The image of the complement contains the complement of the images

up:: Image of Function

Let a function, and .

Let . Then, by definition of a Set Complement,

Thus, .

Note that the reciprocal does not necessarily hold, doesn’t necessarily imply that ─ that is, there can still be some point in that maps to .

Counterexample of the equality

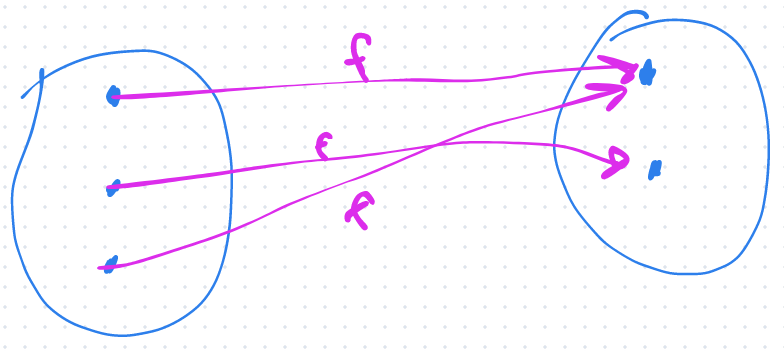
Let , such that .

Let . Then note that

Thus, .

Thus, cases in which distinct points can map to the same point break the equality for images of complements. That is why The injective image of complements is the complement of images.

---

References

• Sutherland, Wilson A. Introduction to metric and topological spaces. Oxford University Press, 2009.